for Tall and Slender Buildings

Virtually all tall buildings are subdivided into zones served by groups of four or six elevators. These groups, with large, single-deck cars, reflect the planning concepts of the attendant-operated elevators of the early 20th century. When attendants were abolished, these groups lost their human intelligence. However, traditional controls with up/down buttons in the lobbies and floor buttons in the cars continued to be the standard group operating system. Without attendants, the performance of groups became erratic and inefficient. This was the reason why, in the past, the use of large cars became a must.

The planning of groups with large cars became habitual. Planners were not aware that large cars are inherently inefficient and a great waste of space and energy. This paper proves that a new zoning concept on the basis of double- or triple-deck cars and intelligent destination controls can reduce the space requirements for groups by up to 50%, compared with those in existing buildings.

Intelligent destination group controls are based on the inherent relativity of group characteristics. For detailed information on this topic, refer to my article “Planning Groups of Elevators for Optimal Performance and Efficiency” (ELEVATOR WORLD, January 2014).

Layered Zoning

Envisage a 30-story building zoned in layers of A and B floors. The two lowest floors are entrance floors connected by escalators, herein identified as AØ and BØ. The 28 upper floors consist of 14 pairs of A and B floors. The lowest pair is identified as floors A1 and B1, the next higher pair as A2 and B2, and so on. All A floors form building zone A, and all B floors form building zone B.

The two zones are served by one group of six double-deck cars. The lower deck of each car is identified with the character A, and the upper deck with B. The A deck of each car serves only A floors and the B deck only B floors. This implies that the maximum number of destinations for the double-deck group is 14.

Floor Distances

The distances between A and B floors are 4 m and match the distance between the A and B decks of the cars. The floor distances between a B and an A floor above may vary. This article will not consider alternatives for double-deck cars that can adjust the distance between their decks.

Performance During Heaviest Simultaneous Up and Down Traffic

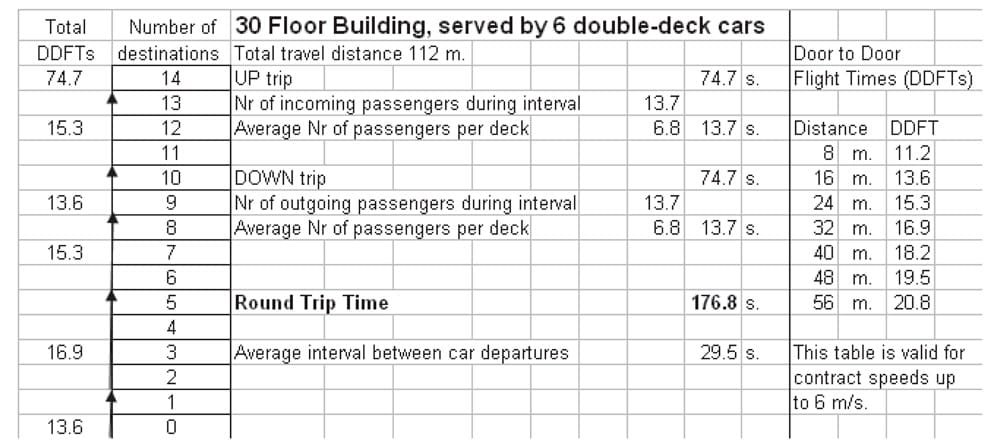
This article evaluates the performance of the six-car double-deck group previously outlined for simultaneous up and down traffic of 7% of the population per 5 min. These traffic conditions are extreme and unlikely to occur in a real building. We assume the following characteristics:

• Travel distance AØ to A14 and BØ to B14: 112 m (14 X 8 m)
• Contract speed: 6 mps
• Contract load: 800 kg per deck
• Population: 1,960 persons (28 X 70)

During the assumed traffic conditions, intelligent destination controls will permit service to only five destinations for up trips, including the top or reversal destination. During down trips from the top/reversal destination, cars serve four more destinations before arrival at destination zero.

On the basis of the permitted numbers of stops, average round-trip times (RTTs) will be 177 s., with an average interval between departures of 30 s. (Table 1). The average car-deck load will be approximately 6.8 passengers.

The time required for a typical up trip is the sum of five door-to-door flight times (DDFTs), plus the assumed time costs for boarding and disembarkation of the average number of 6.8 passengers on each car deck. Down trips will be just as long. The departure times from floor zero; the top floor; and, in fact, all floors, are controlled and coordinated by the intelligent destination control. These controls operate on the basis of timetables for each car and each round trip. Consequently, all time-dependent service qualities are predictable and highly consistent.

Number of Up Passengers and Probable Number of Destinations

During a period of 5 min. with an up traffic density of 7%, the number of passengers entering the building will be 137 (7 X 19.6). During an interval of 30 s., the number of incoming passengers is only 13.7 (137/300 X 30). The mathematical formula for the probable number of destinations of a group of 13.7 passengers that may go to 14 destinations returns the number 8.8. The first departing car serves five destinations, implying that 57% of the incoming group (i.e., 7.8 passengers) can be assigned to the decks of the first departing car on the basis of “first come, first served” until the number of permitted stops is exhausted. The average waiting time (AWT) for these passengers will be approximately 15 s. The remaining 43% of the passengers (i.e., 5.9 passengers on two entrance floors) may have a maximum of nine destinations. Their probable number of destinations is 4.4, which implies all can be assigned to the second next departing car. These passengers will have to await the departure of the first car, plus 30 s. more until the departure of the second car. For these passengers, the AWT is approximately 38 s. (interval X 1.25). The AWT for all passengers will be approximately 25 s. The longest waiting time (i.e., the waiting time bandwidth) will be approximately 45 s. (interval X 1.5).

During the next interval, another group of 13.7 incoming passengers will again produce a subgroup of 43% that will be assigned to the second next-departing car. Obviously, the average car deck load will soon be approximately 6.8 persons.

Service Qualities for Down Passengers

The assignment of down passengers to specific cars is also based on “first come, first served” until the number of permitted stops is exhausted. Down calls that cannot be assigned to the first available car will be assigned to the second available car. Assigning a down or up going passenger at a permitted stop to a car going in the wrong direction but scheduled to reverse direction at the next stop can be used to enhance group efficiency. We may confidently assume that down passengers will not experience waiting and travel times worse than those of incoming passengers.

Conservative Calculation Method

The calculation method of this evaluation is conservative, because it assumes that all trips reverse at the top destination. The mathematical formula for probable destinations assumes that all floor populations and their working hours are identical. In a real building, this will not be true; consequently, the real numbers for probable destinations are likely to be lower than the theoretical numbers. The assumed times for door opening and closing are 4.5 s. and 2 s. for the entry and exit of each passenger. For smaller cars and doors, these times will be shorter.

Interfloor Traffic

Direct trips between destinations are possible if target destinations are already scheduled as permitted destinations or are still available as permitted destinations. If a direct trip is not possible, an interfloor passenger will be informed of the assigned car, as usual. In this case, the trip to the target floor will usually be a trip in the assigned car via floor zero for a down car, or via the reversal floor for an up car. Note that in multitenant buildings, interfloor traffic is very small. During periods with extreme traffic densities, it may be assumed to be negligible. During moderate traffic conditions, flexibility of the number of permitted destinations and direct communication with all passengers via mobile phones will ensure efficient interfloor traffic.

Performance if Permitted Destinations Are Increased to Six

If the number of permitted destinations is increased to six, all performance data will be worse. The average RTT increases to 200 s., and the average car deck loads to 7.6 passengers. The AWT increases by more than 1 s., and the bandwidth increases by 5 s. The most significant change is the 10-s. increase of the longest passenger travel times in the cars to reversal floors. This negatively affects the average time to destination for all passengers with an increase of 5 s.

Performance During Moderate Traffic Conditions

“Moderate traffic conditions” are defined as simultaneous up and down traffic densities of 3% of the population per 5 min. In a real building, traffic densities are likely to be less during the majority of operating hours.

During the assumed traffic conditions, intelligent destination controls may permit service to four destinations during up and down trips. On the basis of this number of permitted stops, average RTTs will be 136 s., and the average interval between departures will be 23 s. The average number of incoming passengers per interval is approximately 4.5 passengers, and their probable number of destinations is four. Consequently, all passengers can be assigned to the decks of the first departing car. Car deck loads will be approximately 2.3 passengers. AWTs for all passengers will be approximately 11 s. Incoming passengers may not distribute equally over both entrance floors, but these variations will not affect group efficiency.

Group service qualities improve if the number of permitted destinations is reduced from four to three, because the average RTT is reduced to 112 s., and departure intervals are reduced to 19 s. Average car deck loads are reduced to 1.8 passengers. The AWT for all passengers is reduced to approximately 10 s. The percentage of incoming passengers that can be assigned to the first departing car is reduced to slightly less than 100%. The most significant change is the reduction of the longest passenger travel times in the cars to reversal destinations by 12 s. This implies that, on average, passengers arrive at their destinations approximately 6 s. earlier. These data are an indication of the flexibility and performance reserves of groups with intelligent destination controls. They also explain why and how building managers can influence the service qualities of intelligent groups.

Performance During Up- or Down-Peak Traffic

The six-car double-deck group can service up-peak traffic densities of 12% by permitting service to only four upper floors during up trips. Down trips will be nonstop (30 s.). This results in average RTTs of 110 s., average car deck loads of 7 persons and AWTs of 20 s. The waiting-time bandwidth will be approximately 42 s., because, theoretically, two passengers (one per deck) arriving during a specific interval may have to be assigned to the third departing car. The few down passengers (typical for up-peak conditions) will be assigned to upward cars. For down-peak traffic, the same methods will produce the same results.

Building Volume Savings/Footprint Reductions

Hoistways usually occupy the full height of a tall building. For this reason, comparisons of the footprints of alternative configurations are good indicators of their space requirements.

Existing 30-floor buildings are usually served by a low- and high-rise group of four cars, each with a contract load of 1600 kg. Their net internal hoistway area is approximately 28 m2 per group. Their lobbies and hoistway enclosures may double their floor-space requirements to 56 m2 per floor and per group. For two groups, their maximum total floor-space requirement may be 2 X 56 X 30 = 3,360 m2. On floors without entrances, lobby space may be reduced to corridor space, a savings of possibly 14 m2 per floor (i.e., on 28 floors, a total of 392 m2). In this case, their total floor-space requirement is reduced to 2,968 m2.

The net internal hoistway area of the six-car double-deck group is also 28 m2. Including lobbies and enclosures. Its total floor space requirement may be as high as 56 m2. The total floor-space requirement of this group would be 30 X 56 = 1,680 m2. Thus, the additional floor space that results from the application of intelligent destination controls and Layered Zoning for the 30-floor building of this study is 2,968 – 1,680 = 1,288 m2. These floor-space gains increase exponentially with the height of a building, because hoistways for additional upper floors reduce the rentable areas of lower floors. Additional footprint savings for groups with intelligent destination controls may be possible on account of smaller lobbies in view of few waiting passengers and in-line configurations.

Traffic Simulation and Contractual Performance Guarantees

Until now, traffic simulation was used to disclose performance parameters of specific groups with proprietary group controls for assumed traffic conditions. In the future, the role of traffic simulation will be very different, because the time-dependent service qualities and the up/down transportation capacities of groups with intelligent destination controls are predictable for any combination of up/down traffic densities. This implies group service qualities can be contractually guaranteed.

Consequently intelligent groups do not require traffic simulation to disclose performance data. However, traffic simulation can be used for independent confirmation of calculated service qualities during the building planning phase. Guarantees of service qualities for a range of defined traffic densities are likely to become a standard element of future elevator contracts. These methods also imply that traffic simulation can be used to check the functionality of intelligent group controls. If traffic simulation does not deliver the calculated/predicted performance data, something is wrong in the control system.

Groups with Multi-Deck Cars

Future buildings may be served by groups with eight or more multi-deck cars that serve many floors with a paternoster-like mode of car operation. These groups may be seen as a chain of cars connected by a software string, instead of roller chains. During heavy traffic, the string of cars rotates faster, because the cars make fewer stops. These groups will feature very short intervals, and waiting and travel times. The cars can be small, because the number of passengers per deck will be very low. The vertical traffic experience will be very different, compared to that of existing buildings. These configurations could be a topic for another article.

 Note that multi-deck cars also permit tall buildings on small building sites. Apartment buildings served by two or three double- or triple-deck cars will be practical and economical.

Conclusions

The discovery of the inherent relativity of group characteristics has been a shock for the author, and this may wel apply for many others in the elevator industry. Particularly for those of us who tried to solve this problem with complicated algorithms, traffic calculations, traffic analyses and traffic simulation, the question that remains is, “Why was relativity such an elusive problem?” The short answer: the performance of groups of elevators is a four-dimensional problem, in which time is the fourth dimension.

Time “costs” control all aspects of group performance, because each stop causes a great loss of time. Cars must slow down and stop, doors have to open, passengers go in and/or out, doors close, and the car must start and accelerate again. These time costs explain why control of the permitted number of stops controls all group characteristics. This simple fact is the basis of intelligent destination group controls.

Intelligent destination group controls can enhance the role of elevator contractors in the building industry. They are likely to be the providers of intelligent building-management systems. Insecurity in respect of group planning and performance is eliminated. Group configurations will define the maximum height and efficiency of future buildings.

To ignore the innovation potential of intelligent destination controls (i.e., the continued use of group controls that do not control numbers of permitted stops and, consequently, do not facilitate optimal car operations and contractual performance guarantees) is not advisable. Elevators are already the pumping hearts of tall buildings; the author suggests that intelligent groups will become the brains of future intelligent buildings, as well. He would greatly appreciate reactions and questions from readers through EW at editorial@elevatorworld.com.