How Fast Could the Fastest Elevators Be?
Feb 1, 2016
Examining what limits passenger-elevator speed and how faster ones will come to be available
by Dr. Albert So and Ricky Chan
Last year, the first author of this article published “Fastest Elevator – A Competition in High Technology” (ELEVATOR WORLD, September 2014), discussing various technologies developed by international manufacturers to break the speed record, now at 1,200 mpm (though not yet installed), while the previous records were 750 mpm in Yokohama Landmark Tower, 1,010 mpm in Taipei 101 and 1,080 in the Shanghai Tower. The last sentence of the article stated “a revolution in the elevator speed record is inexorable” due to the continuous growth in height of ultra-high-rise buildings around the world, which are targeting 1 km tall.
A reader, Pieter J. de Groot, commented (EW, December 2014) that the maximum speed of elevators is limited by the rate of change of air pressure that can be tolerated by passengers. It is well known that the atmospheric air pressure decreases at a rate of approximately 12 millibar (mb) per 100 m, as mentioned in de Groot’s comment. He further pointed out that the pressurizing of cars, which is an undesirable complication but with minimum time benefits, does not solve the problem of reducing the rate of change of air pressure for passengers. This is because control of the in-car air pressure to maintain a constant rate of change requires both depressurizing during the first half of an upward trip, then pressurizing during the second half of the upward trip.
The author response, also in the latter EW issue, stated the manufacturer of the super-high-speed elevators serving Taipei 101 got a controlled rate of change of in-car air pressure at 1.26 mb per second (mbps), which is acceptable to most passengers. As a matter of fact, the particular elevator car at Taipei 101 is airtight, and has an in-car barometer under continuous and complicated control by a blower. In other words, the passengers should feel comfortable when riding in a super-fast elevator car, whatever the speed, provided that the rate of change of in-car pressure is under control. This article will continue the author response by explaining the physics behind the answer, then introduce a potential solution for manufacturers to continuously break speed records.
The Speed Limit Based on Physics of Air Pressure Variations
The actual rate of change of air pressure that limits elevator speed is the pressure external to the hoistway, not inside the car. Though some cars are air sealed, the existing hoistway and building interior are not. That means the air pressure outside the hoistway will drop at a rate of 0.12 mb per meter upward. Even though the car itself can maintain a constant and comfortable rate of air-pressure reduction at 1.26 mbps, the air pressure outside does not drop at the same rate if the elevator speed is too high.
Let us take an extreme example of a car speed of say, 30 mps. In 33 s., the car has already traveled upward approximately 1,000 m with a comfortable air-pressure reduction of 1.26 X 33 = 41.6 mb in total (without the consideration of acceleration and deceleration). However, outside the car, the total air-pressure reduction is equal to 1,000 X 0.12 = 120 mb. When the car stops at the 1,000 m landing and the car/landing doors are opened, the passengers face an immediate air-pressure drop of 120 – 41.6 = 78.4 mb, which is not tolerable by most passengers. It should be noted that the exact change should be much less, because time for acceleration and deceleration is not considered here (though it will be later).
It seems that the comfortable 1.26 mb drop or rise per second and 0.12 mb change of external atmospheric pressure per meter of vertical rise have already limited the car speed to 1.26/0.12 = 10.5 mps. But, the Taipei 101 elevators have broken this limit for more than a decade. This is because we need to address all three types of motion: acceleration, rated-speed operation and deceleration.
The following mathematical model could help us understand more about the whole event and theoretically estimate the speed limit. Figure 1 shows the speed/time curve of a typical elevator car. Zone I is the acceleration region at a mps2, from t = 0 s. to t = t1 s.; Zone II is the rated speed region, at speed = v mps, from t = t1 s. to t2 s.; Zone III is the deceleration region, at –a mps2, from t = t2 s. to t = t2 + t1 s. due to the symmetry of the speed profile. Here, for the sake of simplicity, the very short jerking periods are ignored. Table 1 shows values of relevant kinematic parameters.
The shortest time taken by this typical trip is, of course, when t1 = t2 (i.e., no elongated rated-speed operation, making pressure control most difficult). Then, total time taken = 2 t1 s. and total distance traveled = a (t1)2 m. The total time taken governs the maximum in-car air-pressure change during the whole trip = 2 t1 X 1.26 mb = 2.52 t1 mb. The total distance traveled governs the out-of-car air-pressure change during the whole trip = a (t1)2 X 0.12 mb = 0.12 a (t1)2 mb. To make sure the passengers do not face a sudden drop in air pressure when they exit the car, the inequality represented in Equation 1 is necessary.
0.12at2 < 2.52t1 at1 < 21
(Equation 1)
It should be noted from Table 1 that at1 is also the speed attained, which implies that the maximum speed attained is 21 mps. If the normal acceleration/deceleration rate of 1 mps2 (tolerable by most passengers) is used, t1 must not be longer than 21 s. If another acceleration/deceleration rate is used, t1 will also be different, but the theoretical speed limit is always equal to 21 mps.
It seems that the elevator system that is going to serve the Guangzhou CTF Finance Centre has already reached the extreme. Of course, there is still some room for a slight increase beyond 21 mps by buying time. For example, when the doors are closed at the ground floor, air-pressure reduction can commence while it may take a few more seconds before the car starts to rise. When the car reaches the top floor, it may take a few more seconds for leveling, during which further in-car air-pressure reduction can be achieved. The next section will explain a potential solution to further reduce this pressure, which may allow international elevator manufacturers to continue their speed competition.
Aerodynamic Control of In-Building Air Pressure
As stated in the aforementioned comment, infiltration is highly undesirable in modern buildings when sustainability and energy savings are concerned.[2] When outdoor air uncontrollably leaks into the building through small gaps on the façade (including the fenestration), the cooling or heating load will be greatly increased. Normal engineering guidelines state that all outdoor air must be introduced into a building through filters to remove dust and bacteria, and properly extracted to remove indoor odor. That explains why building interiors are usually slightly pressurized – to make sure only indoor air leaks out, while outdoor air cannot leak in. In other words, it is desirable and possible to control the air pressure of the building interior by using mechanical ventilation for the purpose of saving energy. Sealing up all gaps on the façade would surely help.
An article on high-speed elevators serving Taipei 101[3] mentioned that whenever the atmospheric pressure changes inside or outside the elevator car, it would cause physiological discomfort to the passengers. The author reported that these elevators traveled at contract speeds of 1,010 mpm (16.8 mps) up and 600 mpm (10 mps) down over 382.2 m. The atmospheric pressure difference between the dispatching and destination floors in Taipei 101 is approximately 48 mb. It is well known that any sudden change of atmospheric pressure would normally have made some passengers feel quite uncomfortable or sick, just like in an airplane before landing. To solve this problem, Toshiba developed an atmospheric pressure regulating system to mitigate the discomfort due to difference in pressure inside and outside the car when it is traveling at high speed.
The same principle as that used in Taipei 101’s case can be applied to the study of the hypothetical 1,000-m-tall building, which was proposed in this article’s previous section to be equipped with a super-high-speed elevator trying to run at an imaginary high contract speed of, say, 30 mps. By using this assumption and the natural pressure gradient of 12 mb per 100 m, the total pressure difference between the roof and ground floor is 120 mb. As demonstrated in the previous section and in Taipei 101’s case, a pattern with the rate of in-car pressure change fixed at the same value from start to stop is the most wanted. In its case, the in-car pressure change in an ascending car could be sharply reduced to 1.29 mbps and also reduced during the descent to only 0.96 mbps,[3] which were well within the comfortable tolerance argued so far. These figures were achieved mainly due to a high-pressure blower generating the necessary pressure within the car. In the Taipei 101 case, the aerodynamic shape of the car capsule was also mentioned, while the first author of this article studied the car shape for enhancement of the aerodynamic performance of high-speed elevators.[4]
To address de Groot’s main issue, when the car stops at the 1,000-m-high landing point and the car landing doors are opened, the passengers need to face an immediate air-pressure drop of tens of millibars, which is not tolerable by most passengers. A related study by the authors of this article has since pinpointed this sudden air-pressure drop when at the tallest point of the elevator and corresponding levels below. The study includes a series of calculations on how much added pressure would be required to negate the pressure difference at different heights and levels of this building with the lift car shuttling at 30 mps.
Reference is made to Table 2 showing the changes in time, height, speed, in-car pressure, outdoor atmospheric pressure and indoor pressure to be added for the captioned car traveling from ground floor to top floor at 990 m high with a constant acceleration and deceleration rate of 1 mps2. Here, a uniform floor height of 4 m per floor is assumed. It can be seen that the whole journey takes 63 s. without the consideration of jerking, and that the highest added pressure of approximately 46 mb occurs at a height of around 930-940 m above ground toward the end of the journey. When the added pressure is negative, the indoor air pressure has to be lower than the atmospheric pressure at the same height, and vice versa for a positive added pressure.
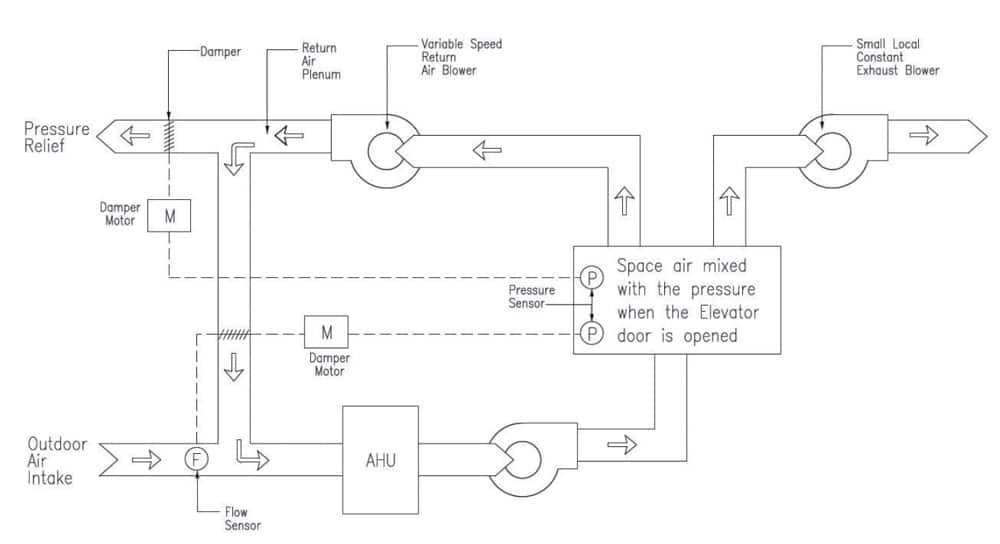
To eliminate the effect of a sudden pressure drop as experienced by passengers when a super-high-speed elevator car lands and opens the doors at every floor level, pressurization of the office building at different levels has to be properly controlled as illustrated in Figure 2 so as to balance out the drastic pressure difference between the elevator car and lobby.
This system applies a high-head fan to generate high air pressure at each floor whenever the required added pressure is positive (as shown in Table 2) to suit the calculated figures based on a 1.26-mb drop per second of travel and a vertical reduction of 0.12 mb per m. Of course, the outdoor atmospheric pressure varies because of various factors, such as instantaneous weather conditions, seasons of the year and the temperature setting of the centralized air-conditioning system. But, all such data can be fed to the building-management system, then the in-car air-pressurization system for dynamic control of the indoor air pressure of every floor. This control is facilitated by standard air-handling systems as shown in Figure 3. The mathematical theory and algorithms for selecting the fans by which to achieve the precise pressure compensation as described above can also be obtained from and referred to in BS 5588-4:1998 or the latest British Standard or European Norm corresponding to staircase pressurization and depressurization.[1]
Analysis
Though this method seems to be a potential solution for the sake of sustainability, in addition to elevator riding comfort, there are technical considerations. Although it is possible to pressurize every floor, it is impossible to pressurize the lift shaft that extends from the ground floor to the top floor. When car/landing doors open at a particular floor, gaps around door edges must first be sealed up so the car is only open to the lobby, instead of the lift shaft. Pressure over the whole floor, including the lobby, must be uniform. In other words, the car, lobby and the whole floor must be airtight against the lift shaft. Cracks on the building façade and around fenestrations must be sealed, or the air leakage rate will be rather high at higher floors, as there is an increasing pressure difference in tens of millibars. Again, the stairways act like the lift shaft and, therefore, the air-pressure variation along the stairways is equivalent to that of the outdoors. Stairways have to be pressurized, as well, as the trend of using elevators for fire escape is maturing, diminishing the reliance on stairways.
It is expected, though not estimated by your authors, that energy consumed to create such an airtight super-high-rise building could be substantial. Fortunately, the pressure difference between consecutive floors as interpolated between the values listed in Table 2 is not so high that air leakage between floors would not be a major concern. For short travels, time spent on acceleration and deceleration is relatively long; therefore, in-car pressure control is less demanding where the 1.26 mbps drop could always be fulfilled. The real challenge lies in a shuttle trip from the ground floor to the top floor of a building almost 1 km tall.
Conclusion
This article argues that it is technically possible for elevators to break the theoretical speed limit, say 21 mps as estimated, by using advanced heating, ventilation and air-conditioning pressurization control. However, the authors agree with de Groot’s view, in general, that further competition on elevator-speed record breaking is not that meaningful, while there are many other ways to tackle shuttle journeys inside super-high-rise buildings. The establishment of shuttle terminals at different heights provides an opportunity for passengers to get used to the lower air pressure at a particular terminal before they proceed to change cars to get to a higher terminal. Another way is to establish a pressure-adaptation lobby at each high landing floor so passengers take some time to stay there before they are allowed to walk into the core of the building. This method has been used by deep-sea divers for years. As announced, the next speed record will be 20 mps. Let us see how far international manufacturers can go!
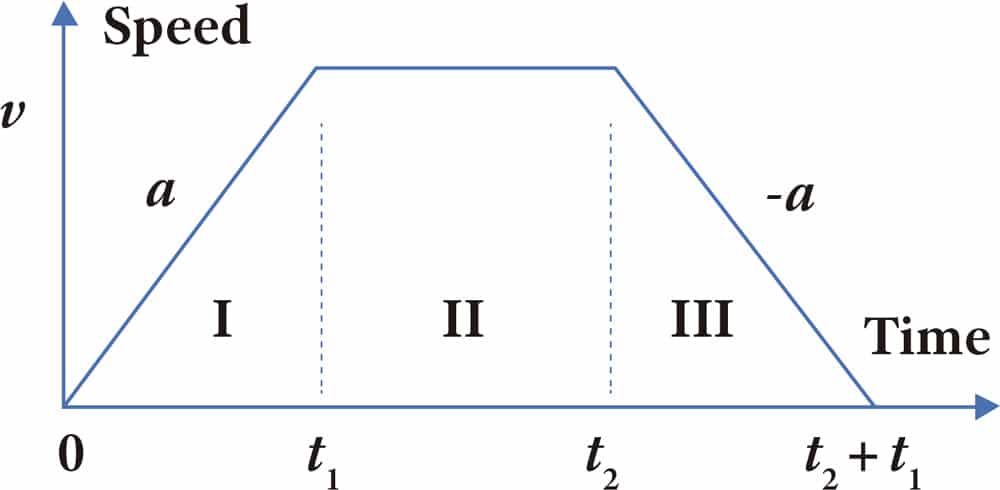
Figure 1: Typical speed/time curve of an elevator car 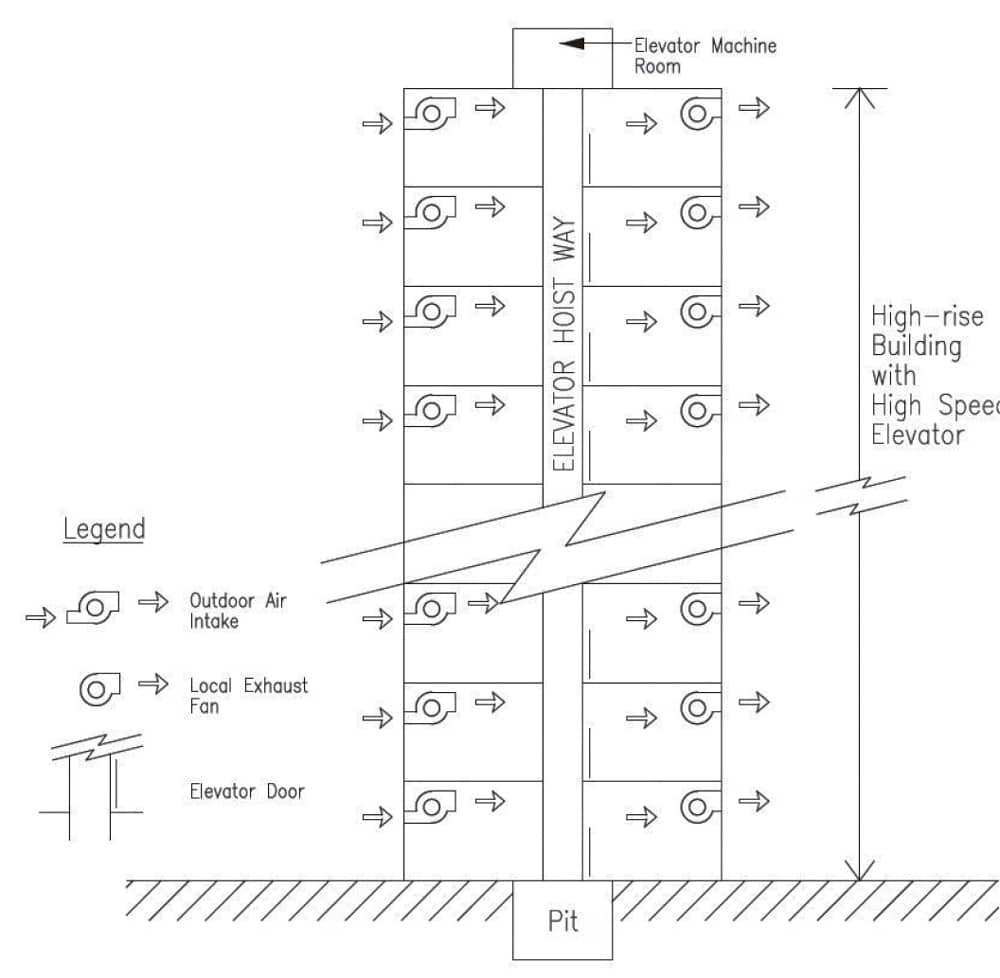
Figure 2: Fan operation on building pressure 
Figure 3: Typical floor air-pressure control system with blowers (detail of Figure 2) 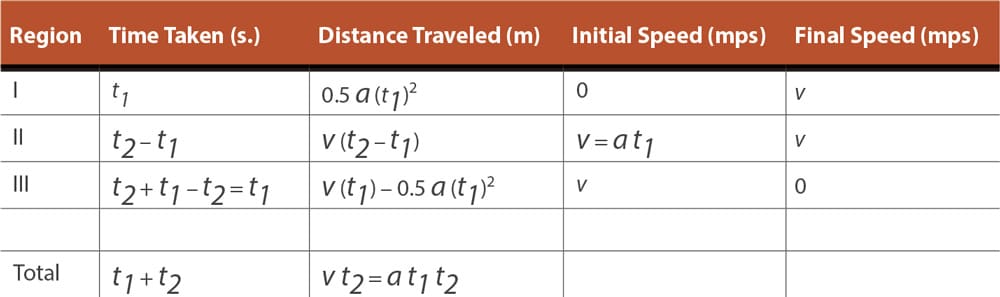
Table 1: Relevant kinematic parameters 
Table 2: Variations of time, height, floor number, location and added air pressure
References
[1] BS 5588-4 (1998): Fire Precautions in the Design, Construction and Use of Buildings – Part 4: Code of Practice for Smoke Control Using Pressure Differentials.
[2] de Groot, P.J. “Comments” and So, A. “Author Response,” ELEVATOR WORLD, December 2014.
[3] Mizuguchi H., et al. “Breaking the 1000 mpm Barrier: High-Speed Elevators in Taipei 101,” EW, September 2005.
[4] So, A. “Fastest Elevator – A Competition in High Technology”, EW, September 2014.
[5] Trane Engineers Newsletter, Managing the ins and outs of Commercial Building Pressurization, American Standard Inc., Vol. 31, No. 2. (2002).
Get more of Elevator World. Sign up for our free e-newsletter.