Study on a Vibration Reduction System for Lift Roller Guides

A design that prioritizes compatibility and improves existing passive roller guides to reduce vibration levels at low cost

This paper was first presented at the 11th Symposium on Lift & Escalator Technologies (liftsymposium.org).

The maintenance and replacement of lift roller guides require time and effort. So, if they can be extended in life, advantages such as reduced maintenance frequency, cost reduction and improved reliability can be obtained. In this research, your authors propose roller guides that achieve long life by changing the material of the roller to provide both ride comfort and durability. This article will show how to decrease lift cabin vibration via constructing an analytical model for calculating the time history response of the lift cabin and verifying the vibration control effect by simulation incorporating spring and damping elements based on the experimental results. Through analytical and experimental approaches, a design for a roller guide with optimal riding comfort and durability within the range of safety regulations will be proposed.

Introduction

The number of lifts has been increasing in recent years, due to facility investment for construction of high-rise buildings by redevelopment in urban areas, barrier-free initiatives and modernization and replacement needs. Demand will likely increase further due to the impact of emerging countries and redevelopment. Roller guides form part of the car-frame guiding system and the elastic-damping properties of the roller guide shoe (wheel tire). If included, additional spring-damper elements provide passive vibration control via a mechanism installed between the rail in the lift shaft and cabin on which the person rides and plays the role of smoothly moving up and down, while suppressing vibration. 

There is a shortage of human resources responsible for lift maintenance. Since roller guides require maintenance and replacement, it is considered meaningful to extend their service life (durability) to reduce maintenance frequency, cost and reliability. If the rollers are hardened to increase durability, comfort and noise will deteriorate. In this study, the problem of vibration and abrasion in the roller guides is focused and aimed at examining the specification conditions of the roller guides to achieve both comfort and durability. Specifically, experiments to investigate the mechanical properties and durability of the roller guides are conducted.

Roller Guides

Much of the vibration in a moving cabin is attributed to the distortion of the guide rail installed in the lift shaft. Figure 1 shows the cab structure. The main cause of distortion is low accuracy during installation^[1] or distortion due to age,^[2] and existing lifts require drastic renovation for improvement. The distortion of the guide rail propagates inside the cabin, along the roller guides, frame and rubber mounts. Figure 2 shows the actual roller guides used for the experimental and analytical investigations.

The roller guides handled here are composed of a coil spring, control arm and circular roller. The material of the roller part is natural rubber, but it cracks and eventually peels off due to wear. It is common to thoroughly implement preventive replacement to increase hardness and durability as a countermeasure.

This research focuses on roller guide vibration and durability problems. After replacing the material of the roller part to secure durability, the specification conditions of the roller guide compatible with riding comfort is examined. A roller guide design that achieves both optimum riding comfort and durability, while meeting safety regulations, can be created through analytical and experimental processes. Active roller guides are effective in significantly suppressing vibration. Manufacturers are developing such guides, but they are costly and difficult adjust.^[3]

In this study, the roller guide system that prioritizes compatibility and improves existing passive roller guides to reduce vibration levels at low cost is developed. In the research, the vibration level at which passengers do not feel uncomfortable is root mean square (RMS) acceleration of 0.1 m/s².^[4] It also sets a displacement as small as possible without making contact with other structures as an allowable limit. The roller guides used in this study have low flatness (Figure 2). Since a change in mechanical characteristics due to displacement is expected, a loading test using a hydraulic servo actuator was conducted. Furthermore, the urethane elastomer roller with a hardness of the JIS-A standard 95° has excellent wear resistance. (This material is also used in roller guides for public-transportation-system railroad tracks, automated guideway transit systems and roller coasters.) This roller was prototyped, and the same experiment was performed.

Analysis of Car Vibration

Analytical Model

An analytical model that imitates an actual six-person ride machine was constructed to calculate the lateral vibration of the running lift.^[5] In this model, the vibration transmitted from the rail roughness to the cabin via the roller guide, coil spring, frame and the rubber mounts^[6] are calculated. An eight-degrees-of-freedom (8-DOF) analytical model was constructed to calculate the horizontal movement of the four rollers and the horizontal movement and rotation of the frame and cabin. The center of rotation is the same for the frame and the cabin. Waves of the same waveform are input to the upper and lower rollers with a time difference. Acceleration/deceleration of the lift was not included. Figure 3 shows the 8-DOF analytical model. MATLAB2020a was used for the analysis. Figure 4 shows an overview of the model.

Equations 1-8 show this model’s equation of motion. Variables used are:

• Un: input displacement

• krn: stiffness of roller

• crn: damping coefficient of roller

• mr: mass of roller

• xrn: displacement of roller

• n: each number (1-4)

• ks: stiffness of coil spring

• cs: damping coefficient attaching to coil spring

• k1: stiffness of upper rubber mount

• c1: damping coefficient of upper rubber mount

• k2: stiffness of lower rubber mount

• c2: damping coefficient of lower rubber mount

• m: mass of frame

• xf: displacement of frame

• f: rotation of frame

• Jf: Moment of inertia in frame

• w1 and w2: width of frame

• h1 and h2: height of frame

• M: mass of cabin

• x: displacement of cabin

• θ: rotation of cabin

• J: moment of inertia in cabin

• W1 and W2: width of cabin

• H1 and H2: height of cabin

In the actual response analysis, the following variables are substituted for the above variables: m = 1200 kg, w1 =0.9 m, w2 = 0.9 m, h1 = 1.5 m, h2 = 1.5 m, M =1000 kg, W1 =0.75 m, W2 = 0.75 m, H1 = 1.25 m, H2 = 1.25 mm, k1 = 400 N/mm, k2 = 400 N/mm, k2z = 2000 N/mm , c1 = 400 N･s/mm, and c2 = 400 N･s/mm.

Modeling Roller Guide Mechanical Characteristics 

The response displacement of the commonly used rubber roller and prototyped urethane roller with increased hardness, the stiffness and the damping coefficient dependence on the vibration frequency and displacement are investigated in the following experiments. Mechanical characteristics for the roller guides in a loading experiment^{[7 & 8]} using a hydraulic servo actuator are investigated, and an operation test using a miniature test apparatus is evaluated. Figures 5 and 6 show the results. The hardness and damping coefficients of the urethane roller are about three times those of the rubber roller. In addition, the amplitude dependence, which is a characteristic of urethane elastomer with hardness of JIS-A standard 95°, was confirmed. 

The dependency functions for mechanical characteristics of roller guides in amplitude dependency and frequency dependency are obtained from the experiment’s results. Eq. 9-12 shows the mechanical characteristics for the roller guides. These formulae are derived from the amplitude dependency and frequency dependency obtained from the loading test of the roller guide. Therefore, in this formula, if the arbitrary frequency and amplitude are substituted as variables, the stiffness and damping coefficient under an arbitrary condition are calculated. Here, xi = displacement of input wave in mm, and fi = vibration frequency in Hz.

Analytical Result

The vibration-reduction effect of the cab is verified using the analytical model introduced above. Although the running speed is set to be arbitrary in the analytical model, this time it was set to 105 m/min as a medium speed. The running speed affects rail bends and gap interval. Figure 7 shows the input waveform. 

It is difficult to measure the roughness of the rail. Instead, the synthetic wave was used to simulate various irregularities of roughness on the rail. Since the main component of the waveform is 5 Hz on the left and 2 Hz on the right, the parameter fi that affects the stiffness and damping of the roller is adjusted to these dominant frequencies. The parameter xi changes moment by moment according to the displacement of input. The maximum displacement of rail roughness is 0.1 mm. The wave inputs to the upper, lower, left and right rollers. Furthermore, a sinusoidal wave simulating a maximum rail distortion of 3 mm was synthesized on one side, and the 0.5-mm gap was synthesized on both sides. Acceleration on the cabin floor, ; displacement, x, and rotation, , of the cab are obtained by response analysis. 

Figure 8 shows the vibration waveform when a rubber roller and a urethane roller are installed. The installation of urethane rollers improves the strength more than rubber rollers do, but the riding comfort may deteriorate, so it is necessary to consider the concept of damping the entire system. The periodic fluctuations of displacement and rotation are the effects of rail distortion. In the next step, a coil spring is installed between the roller and cabin to reduce response vibration. 

Figures 9 and 10 show the verification of the optimum mechanical characteristics and the vibration waveform over time. The maximum response of the coil spring stiffness was set to be less than that of the roller stiffness. Under the condition, the stiffness that satisfies the RMS acceleration of 0.1 m/s² is in the range of 800 N/mm. It was attempted to reduce vibration by substantially adding damping to the coil spring. As a result, reduction of RMS acceleration was confirmed with a damping ratio of 7.5%. Table 1 compares RMS and maximum acceleration under each condition. RMS acceleration when using the rubber roller was set to 100% and expressed as the vibration reduction level. As a result, RMS acceleration was reduced by up to 60% by adding the coil spring under the analysis conditions of this time.

Driving Experiment Using Miniature Test System 

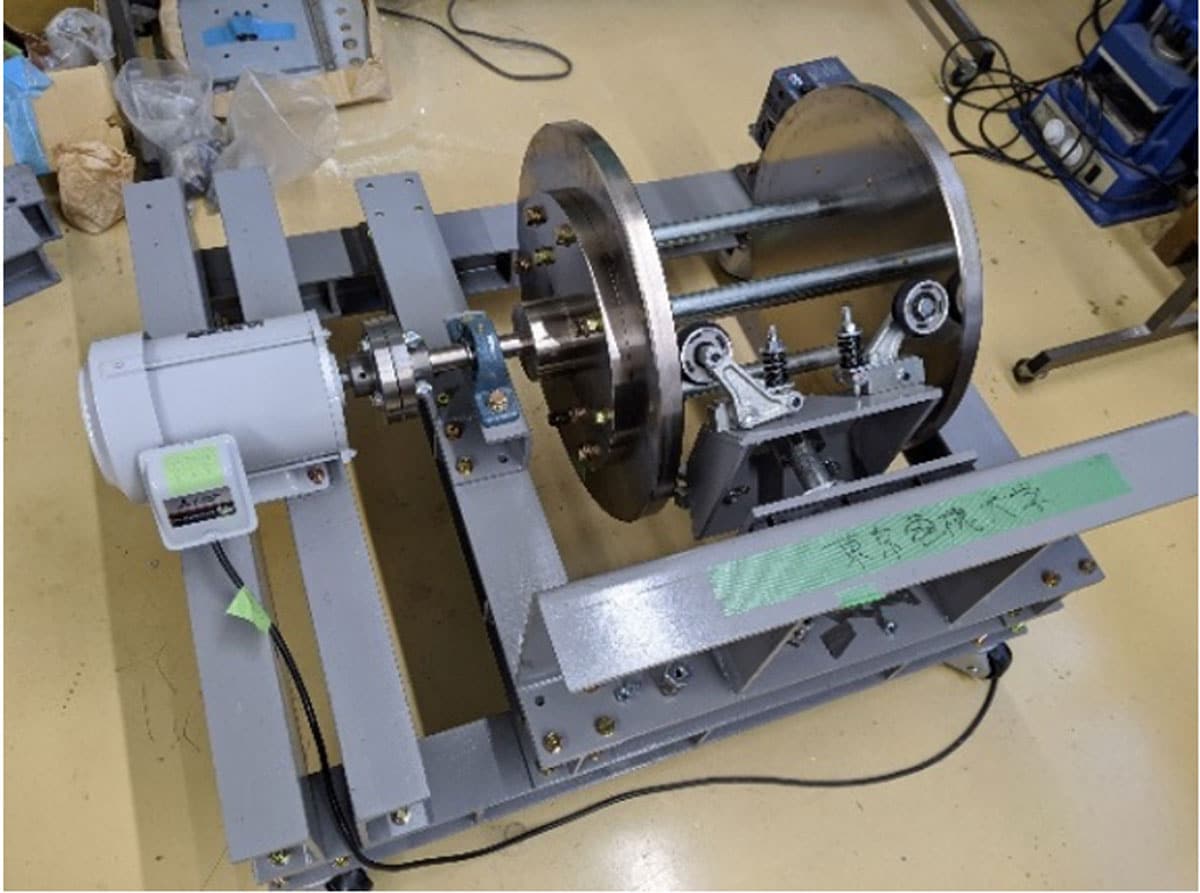
The experiment using the actual lift is expensive and time-consuming, and it is not realistic to repeat the durability test. In this study, the reduction tester for roller guides was made, and a running test was conducted. The experiment equipment consists of a disk that imitates the rail roughness and an iron plate that imitates the cabin. The size of this equipment is 1129 X 930 X 710 mm. The horizontal vibration of the cabin and durability of the roller are verified by attaching a roller guide to a disk and running it. Figure 12 shows the overview of the experiment equipment. Vibration transmission when overcoming the projection is simulated by attaching 10-mm-wide shim tape to the disk and running it. The thickness of one piece of shim tape is 0.1 mm, and any number of these can be stacked. The cabin can rotate freely in the horizontal and rotational directions by means of linear guides and bearings. An accelerometer (KYOWA AS2GB) and data recorder (TEAC es8) were attached to the center of the cabin, and recording was performed at a sampling frequency of 1000 Hz. The analytical model is a combination of the frame and cabin of the model introduced in “Analytical Model” above. Equations (1) to (4) are the same as the model introduced in that section, but the equation of the mass part is different. Figure 11 shows the 6-DOF analytical model. 

Equations (13) and (14) show the equation of motion in the mass part. Parameters used are: m = 15 kg, w1 =0.125 m, w2 = 0.125 m, h1 = 0.115 m, and h2 = 0.115 m:

Figure 13 shows the input waveform and analysis and test results. The waveform that reproduced the protrusion was put into the analysis program for the actual machine and compared with the test results. When comparing the experiment and analysis results, the peak waveform periods are shifted. This is probably because the rotation of the disk is unstable due to insufficient torque of the induction motor on the equipment. Although there are other differences in acceleration levels that are thought to be due to the shim-mounting method, the reproducibility is generally good, and it can be used to develop elements such as rollers and springs. In the future, conducting a verification experiment to verify the optimum value calculated using the analysis model and conducting a durability test of roller guides made of different materials are considered.

Conclusion

A simulation analysis was performed using an 8-DOF analysis model to improve the durability of the roller guides and enhance the vibration reduction effect. As a result, it was confirmed that the vibration can be sufficiently reduced to 87.7% at maximum by using the coil spring and damping, even if the roller hardened to enhance durability is used. The acceleration at this time is much lower than 0.1 m/s², and it is thought that a comfortable ride for passengers can be realized.

This time, the stiffness of the coil spring was calculated within the range of less than 800 N/mm. In general, the weight of the cabin is lighter, and the response tends to be larger when the number of passengers is smaller, versus when the elevator is full. The RMS acceleration is less than the standard 0.1 m/s², even when there is no load. The key point for vibration reduction when using a urethane roller with enhanced durability is the setting of the coil spring. Also, by adding damping, it is possible to further suppress RMS acceleration while maintaining the same maximum acceleration. In the actual lift, the stroke of the coil spring is limited, because the frame does not contact other structures. In consideration of the deviation of the riding position and a disturbance such as an earthquake, the stiffness is set to be larger than the value that can suppress the vibration in the analysis to the greatest extent. In addition, the waveform used in the simulation is a little worse than that expected in the actual machine, but it can sufficiently satisfy ride comfort.

Also, a reduction test device for roller guides was manufactured for the durability test. Here, a rail roughness, including protrusions, was reproduced by placing shims on the surface of the device. Comparing the operation test results with the analytical model simulation results, the reproducibility is generally good, and it can be used to develop elements such as rollers and springs.

A roller guide durability experiment using a reduction tester and verifying the vibration reduction effect of the coil spring with damping will be performed in the future. By installing the spring, it can be expected to greatly reduce both acceleration and displacement. It should be particularly effective in situations in which the rail roughness is bad (rough) as verified by simulation. Also, the response in a driving process from startup to stop, including increase/decrease of load/eccentricity, will be investigated.

Acknowledgements

The 8-DOF analytical model used in the article was constructed in cooperation with Yuya Tase and Reoya Namiki of Tokyo Denki University. The urethane roller used was produced by Ohtsu Chemical Co., Ltd. The reduction tester for the roller guides used was produced by SEC Elevator Co., Ltd. The authors would like to express their appreciation for the support.

References

[1] Satoshi Fujita, Hiroshi Kamaike, Motoo Shimoaki and Keisuke Minagawa. Elevator and Escalator Engineering, p. 156-160, (2019).

[2] Nobuyoshi Mutoh, Kenkichi Kagomiya, Toshiaki Kurosawa, Masahiro Konya and Takeki Andoh. “Horizontal Vibration Suppression Method Suitable for Super-High-Speed Elevators,” Transactions on Electrical and Electronic Engineering, Vol. 118-D, No. 3 (1998).

[3] Naoaki Noguchi, Atsushi Arakawa, Koichi Miyata, Takuya Yoshimura and Seiichi Shin. “Study on Active Vibration Control for High-Speed Elevators,” Journal of System Design and Dynamics, Vol. 5, No. 1, p. 164-179 (2011).

[4] Kiyoshi Funai. “Technologies of Safety and Ride Comfort for Elevators,” International Association of Traffic and Safety Sciences, Vol. 27, No. 2, p. 31-39 (2002).

[5] Hiroyuki Kimura, Mimpei Morishita and Shigeo Nakagaki. “Simulation Techniques for Elevators,” Toshiba Review, Vol. 58, p. 42-45 (2003).

[6] Aritomo Nakano. “Types and properties of anti-vibration materials,” Journal of Environmental Conservation Engineering, Vol. 20, No. 6, p. 400-402 (1991).

[7] Kazuhito Misaji, Hideki Kato and Koichi Shibata. “Vibration Analysis of Rubber Vibration Isolators of Vehicle Using the Restoring Force Model of Power Function Type,” Transactions of the Japan Society of Mechanical Engineers Series C, Vol. 60, No. 578, p. 679-685 (1994).

[8] Satoshi Fujita, Osamu Furuya, Yoji Suizu, Yasuhiro Kasahara, Takayuki Teramoto and Haruyuki Kitamura, “Vibration Control of High-Rise Buildings Using High-Damping Rubber Damper (2nd Report, Loading Tests and Design Formula for Cylinder-Type High-Damping Rubber Damper),” Transactions of the Japan Society of Mechanical Engineers Series C, Vol. 61, No. 585, p. 1885-1890 (1995).

Yosuke Shima is a master’s course student in Mechanical Engineering at Tokyo Denki University. He researches lift cabin vibration reduction.